Composer Peter Kulichkin. Integral Intensity of Artistic Life: Three Approaches

The evolutionary dynamics of various national schools (branches of artistic life) is well-elaborated problem nowadays. But at least one question connected with it was not answered satisfactory: "Do one branches of artistic life develop independently on each other or not?" Let us suppose independence. But how can we interpret synchronism according to cyclic behavior of art style? On the other hand supposing that evolution of one national school depends on evolution of another one make us regard these schools as single whole. So the integral intensity of this whole "school" is expected to exist. But finding a mathematically correct way to measure such integral intensity is very difficult, almost impossible quest. However we would take chances in this field and suggest three approaches to calculate the integral intensity of artistic life.

What does "intensity of artistic life" mean? In line with the existing tradition (Martindale, 1990), the data on a lot of authors are taken out  from an encyclopaedia, authors' years of birth and the length of an article devoted to each author's creative activity (number of lines). All authors are grouped in 10-year intervals depending on their birthdates: 1750-1759, 1760-1769, ... . For each ten-year interval (t) the total NUMBER OF AUTHORS (n) and the total NUMBER OF LINES devoted to them (N) are calculated. The last value (N) is the indicator of the INTENSITY of artistic life (Petrov & Majoul, 2002).

Upon the earlier analysis (Kulichkin, 2004) we realize that:

1. If the number of authors (n) serves as a factor of «popularity» or «prestige» of a particular kind of art in the professional artistic environment, there should be an indicator of «quality of intensity» - the «average mastery». This parameter is SPECIFIC INTENSITY q: q(t)=N(t)/n(t).
2. Changes in the intensity of artistic life have to be regarded as a dynamic process: each generation of authors keeps in mind the experience of the previous generation.
3. There are six versions of three changes in the intensity parameters (n, N and q):
   1. n up, N up, q up - RISE. This way usually describes the creation of a national school. The given kind of art becomes popular in the professional artistic environment, the internal resources and mastery increase. So the potential of the national school is rather high.
   2. n down, N down, q down - DECLINE. If this version of change in the intensity parameters continues for a long time, the potential of the national school is likely to be exhausted. Then, if any sources (internal or external) are not found, the national school disappears surely.
   3. n down, N up, q up - ACCUMULATION. The national school resists «author-replication» and so makes its potential raise. Popularity decreases, but this raise of the potential provides for one more RISE. Usually, the first one has already been before this period.
   4. n up, N down, q down - DISSIPATION. After major achievements of the national school, the popularity of the given kind of art grows rapidly (at the expense of the internal potential exhausting).
   5. n up, N up, q down - EXTERNAL GROWTH. The internal potential of the national school decreases, but the intensity grows. This fact can be explained only by influence of another national schools, kinds of art or other external causes.
   6. n down, N down, q up - EXTERNAL DESTRUCTION. The national school accumulates its internal resources, but popularity of the kind of art declines. So there are some external causes (political, religious, social, cultural, etc.) that refuse the successful development of the national school.

In our research we used the data on 5180 composers of 19 national schools, 610 painters of 4 national schools and borrowed from earlier researches (Petrov & Majoul, 2002) 307 Russian poets and 480 Russian prose writers. Basing on this data, for each national school (of different kinds of art) we can calculate numbers of n, N, q and clear up which version of evolution the national school experience during each ten year interval t. But how can we measure the integral intensity if the data of different national schools is taken out from different encyclopedias?

The first approach to measure the integral intensity we would call VERSION PUZZLE. This approach doesn't require any additional hypothesis, but give us information only about version of changes in the intensity parameters (and no information about the value of the intensity N). For each ten interval t versions of evolution of the intensity parameters n, N and q (related to each given national school) are aggregated. The approach is illustrated by Table 1. Opposite versions are simply struck out. [For example: In 1770-79 (years of birth are meant) Russian literature experienced DECLINE, Russian music - DECLINE too and Russian painting - RISE. Opposite versions RISE and DECLINE may be deleted together out and resultant version is DECLINE]

Table 1. VERSION PUZZLE on three branches of the intensity of artistic life in Russia: literature, music and painting.  

In 5 cases (out of a total 15) we don't deal with any opposite versions (corresponding ten year intervals are marked by asteriska *). The method of VERSION PUZZLE cannot give us exact resultant versions in such cases. The "approximate" versions are on Table 1 in parentheses.

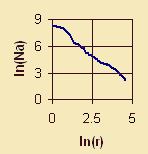
Fig. 1. German music life 1680-1729 (years of birth are meant). The distribution of the number of lines devoted to an author (Na) depending on his rank in the hierarchy of significance (r) [logarithmical coordinates].

The second approach is ZIPF APPROXIMATION. This one requires rather strong hypothesis: the distribution of number of  lines (devoted to authors) must agree with Zipf law (for each national school). If the linear combination of aggregated number of lines agree with Zipf law too - we may regard these national schools as a whole "school". Are there any difficulties? We realize that usually numbers of lines devoted to authors of each national school agree with Zipf distribution (The example is on Fig. 1). But unfortunately at least one notorious artifact take place indeed. We mean the long-term trend (Petrov & Majoul, 2002). This hill-like trend is connected with the procedure of compiling encyclopedias: there is only a few information about authors of remote eras and about contemporary artists as well. It's very difficult to subtract this artifact from the number of the intensity (N) correctly. But without subtracting the trend we may construct linear combinations for current distributions only (using periods not longer than 20-25 years). Finally, regarding short-time intervals we often deal with lack of necessary statistics that makes ZIPF APPROXIMATION difficult to apply.

The last approach is INTER-BRANCH SCALING. It is based on the supposition that the number of lines devoted to authors by encyclopedia compilers doesn't depend on the kind of art. For example, if compilers of musical encyclopedia can "evaluate" painters they would use the same "scale of grades" as they really use for evaluating composers. So we can evaluate painters' significance using the "scale" of musical encyclopedia (or vice versa). Firstly, we arrange composers and painters depending on numbers of lines devoted to them in corresponding encyclopedias. So we have two hierarchies. Then the procedure of SCALING is made: for each painter from "painters' hierarchy" we replace the number of lines (devoted to him in "encyclopedia of painters") by the number of lines devoted to the composer with the corresponding rank in "composers' hierarchy". [For example, let  the "encyclopedia of painting" devote to the most significant painter Xxx 100 lines and the "encyclopedia of music" devote to the most significant composer Yyy 500 lines. We shold assign painter Xxx the number "500 lines"] After that we group painters into ten year intervals and, using "new" numbers of lines, calculate SCALED INTENSITY (N*). The value of INTEGRAL INTENSITY equals the sum of the intensity of music life and the scaled intensity of artistic life related to painting. The example is in Table 2. Nm is the value of the intensity of music life. Np is the value of the intensity of artistic life (related to painting) that is measured in the ordinary way. Np* is the value of SCALED intensity of artistic life (related to painting). It is calculated using the "scale of grades" of musical encyclopedia. N is the value of the integral intensity: N=Nm+Np*.

The analysis comes to the following conclusions. VERSION PUZZLE is effective if a lot of schools (three and more) have to be aggregated into a whole. But it gives us a minimum of qualitative information about the integral intensity. The most "informative" approach - ZIPF APPROXIMATION - demands too strict requirements that make it difficult to be applied. On one hand, INTER-BRANCH SCALING seems to be optimal. But on the other hand, it needs additional theoretical suppositions in each case. We may also realize that despite a lot of differences between examined approaches they all lead to almost similar results, agreeing with earlier researches (Kulichkin, 2005). Thus, the phenomenon of the INTEGRAL INTENSITY is likely to have at least minor empirical confirmation.

Table 2. INTER-BRANCH SCALING for two branches of the intensity of artistic life in France: music and painting.

References

Kulichkin, P. A. (2004). Evolution of artistic life: Russian literature and Russian music in the XIXth century (quantitative approach). In J. P. Frois, P. Andrade & J. F. Marques (Eds.), Art and Science - Proceedings of the XVIII Congress of the International Assotiation of Empirical Aesthetics (pp. 112-115). Lisbon: Calouste Gulbenkian Foundation.

Kulichkin, P.A. (2005). "Evolutionary Genius" and the intensity of artistic life: Italian, French, Austrian and German music in the XVI-XIXth centuries (Quantitative approach). In E. Malianov, C. Martindale, E. Berezina, L. Dorfman, D. Leontiev, V. Petrov & P. Locher (Eds.), Proceedings of the International Congress on Aesthetics, Creativity, and Psychology of the Arts (pp. 219-221). Perm: Administration of Perm Region, Perm State Institute of Art and Culture; Moscow: Smysl.

Martindale, C. (1990). The Clockwork Muse: The Predictability of Artistic Change. New York, NY: Basic Books.

Petrov, V. M. & Majoul, L. A. (2002). Pulsation of literary life: Periodical behavior of Russian poetry and prose in light of the information approach. Rivista di Psicologia dell'Arte, XXIII, 13, 25-40.